The effect of depletion on DFIT pressure and stress measurements
I was asked an interesting question recently – how does depletion affect a DFIT? Often, DFITs are performed in wells with neighbors that have been producing for months or years. The depletion of the neighboring well may impact the DFIT by changing the stress and pressure in the formation.
Pore pressure and stress are closely linked. As reservoir pressure decreases during production, stress correspondingly decreases. A common ‘rule of thumb’ is that stress should decrease by about 2/3-3/4 of the amount that pressure decreases. If we perform a DFIT near a depleted well, can we rely on this 'rule of thumb'? As usual, it is critical to consider the underlying assumptions. The 'rule of thumb' is based on a one-dimensional equation that assumes laterally uniform depletion. What if depletion isn’t uniform?
To investigate, I performed a series of ResFrac simulations. First, I ran a ‘base case’ simulation of a DFIT in a formation with uniform stress and pressure. I chose simulation parameters so that the simulated transient resembles an actual DFIT transient. Next, I performed simulations of several DFIT scenarios: (a) injection into a thin layer of depleted stress and pressure, (b) injection into a layer with high stress and pressure surrounded by layers of depleted stress and pressure, and (c) injection laterally offset from a preexisting hydraulic fracture that is producing into a neighboring well.
In scenarios (a) and (b), the simulated closure pressure and pore pressure was controlled by the depleted zones. In other words, the results were consistent with the idea that stress decrease can be approximately predicted by the ‘rule of thumb’ for poroelastic pressure depletion. One exception was that in scenario (a), if the middle depleted layer was sufficiently thin (and not too depleted), then the fracture grew far out of the middle zone, and the apparent closure pressure was controlled by the non-depleted surrounding zones. But as long as the zone was sufficiently thick and depleted, it controlled the DFIT behavior and the ‘rule of thumb’ held up.
Scenario (c) was the most interesting. Rather than assuming laterally uniform depletion (the assumption in scenarios (a) and (b)), ResFrac calculated the three-dimensional distribution of pressure and poroelastically induced stress around a producing hydraulic fracture from a neighboring well. In this scenario, the 'rule of thumb' overestimated the stress change induced by the pressure depletion. The apparent pressure in the DFIT was consistent with the lower, depleted pressure. But the apparent closure pressure was close to the non-depleted, higher stress. If this seems surprising and counter-intuitive, please read on.
A one-dimensional model of depletion
Where does the 'rule of thumb' come from and is it always reliable? The following equation is often used for estimating the minimum principal stress:

(1)
Sigma_v is the minimum principal stress, nu is Poisson’s ratio, sigma_v is the vertical principal stress, P is pore pressure, alpha is the Biot coefficient, and sigma_t is the tectonic stress. Sometimes, the second pressure term in Equation 1 is multiplied by the Biot coefficient. This equation expresses the idea that the stress comes from three sources: the horizontal squeezing outward of the rock due to the vertical compression, the pressure exerted by the fluid in the surrounding rock, and tectonics. In practice, the tectonic stress term is a fudge factor that cannot be reliably predicted in advance. This is why it is necessary to perform hydraulic tests to directly measure stress, and uncalibrated stress estimates from well logs are unreliable. The greatest value of Equation 1 is that it shows the relationships between variables. For example, it shows that layers with higher Poisson’s ratio have higher stress. Relevant to this discussion, Equation 1 predicts that stress decreases as pressure decreases. If you assume Biot coefficient of 1.0 and Poisson’s ratio of 0.25, you recover the ‘rule of thumb’ prediction that when pressure decreases, stress decreases by 2/3 of the amount of the pressure decrease.
Equation 1 is derived based on the assumption that all parameters are laterally homogeneous. So here is the key question: how are DFIT stress and pressure measurements affected if there is vertical and lateral variability in depletion?
ResFrac Simulations
To investigate, I performed ResFrac simulations of three DFIT scenarios: (1) injection into a layer with depleted pressure and stress relative to the surrounding zones, (2) injection into a layer with high pressure and stress relative to surrounding depleted zones, and (3) injection that is laterally offset from a preexisting hydraulic fracture that is connected to a neighboring producing well.
ResFrac is a 3D, fully coupled hydraulic fracturing, reservoir, and wellbore simulator. It simulates propagation of hydraulic fractures, and realistically describes closure by switching to a nonlinear joint closure law when the walls come into contact. This is similar to the approach used by McClure et al. (2016) in SPE 179725 to perform simulations that closely match realistic DFIT transients. In addition, ResFrac is a fully numerical reservoir simulator that can calculate the stresses induced by pressure depletion.
Baseline simulation
First, I set up a baseline simulation in a formation with uniform pressure and stress trends with depth. All subsequent simulations are modifications of this baseline simulation.
Injection is performed at 2 bpm for four minutes and then at 8 bpm for two minutes. The minimum principal stress is 8300 psi at 10,000 ft, and the gradient in minimum principal stress is 0.85 psi/ft. The initial fluid pressure at 10,000 ft is 5500 psi.
The wellbore is meshed to the surface and has a 10,000 ft vertical section and a 10,000 ft horizontal section, all with 5 inch inner diameter. The reservoir contains oil above the bubble point, with viscosity of 0.3 cp and connate water saturation of 0.2 (in the scenario (c) simulation, I used oil viscosity of 3 cp). Permeability is set to 250 nd, and porosity is set to 5%. The water viscosity is 0.3 cp. Young’s modulus is set to 2e6 psi, and Poisson’s ratio is set to 0.25. The Barton-Bandis joint closure law is used to describe fracture aperture after closure, with aperture at closure equal to 0.01 ft and 90% closure stress equal to 1000 psi. The fracture toughness is set to 3000 psi-in^(1/2). A ‘near wellbore complexity’ rate-dependent pressure drop term is included between the wellbore and the fracture in order to reproduce the rapid drop in fluid pressure after shut-in. A rate-dependent perforation pressure drop term is also included. The fracture toughness increases at a rate proportional to the square root of fracture dimension (either height or length, whichever is smaller). The simulation is isothermal.
The figure below shows the G-function plot from the baseline simulation. The simulated G-function plot is similar to an actual DFIT transient that was shared with me recently by an operator. The G*dP/dG curve begins to bend upward when the fracture mechanically closes at about 8500 psi, a couple hundred psi above the minimum principal stress.

The upward bend in G*dP/dG does not represent height recession or ‘closure of transverse fractures,’ as proposed by some investigators. As shown by McClure et al. (2016) in SPE 179725, the upward bend in G*dP/dG occurs because of increasing fracture stiffness at mechanical closure. The fracture mechanically closes at a pressure a few hundred psi greater than the minimum principal stress because of the stress shadow caused by the residual aperture at closure.
DFIT in a Depleted Layer
Next, I set up a model with a 20 ft thick depleted layer. In this layer, the fluid pressure is 5500 psi. In the surrounding layers, the fluid pressure is 6500 psi. The stress in the depleted layer is decreased 750 psi (to 7550 psi), relative to the surrounding layers (8300 psi). The resulting transient is shown in the figure below.

The fracture closure pressure is about 500 psi lower than in the baseline simulation. The stress in the middle layer is 750 psi lower than the base case. Thus, the surrounding layers appear to have had some effect on the apparent closure pressure, causing it to be a bit higher than would be expected from the stress solely in the middle layer. But clearly, the apparent closure pressure has been significantly reduced by the depletion in the middle layer. The figure below shows the fracture at shut-in. The fracture is predominantly confined within the depleted layer.

I experimented with making the middle layer even thinner and less depleted. In the following simulation, the middle depleted layer is only 8 ft thick and has stress only 300 psi lower than the surrounding formations. In this case, the apparent closure pressure in the transient is controlled by the stress in the surrounding layers and is similar to the baseline simulation. The ResFrac screenshot below shows the fracture at shut-in. The fracture has not remained confined within the middle layer.


DFIT Surrounded by Depleted Layers
Next, I tested the opposite scenario, where the middle layer has higher stress and pressure than the surrounding formations. In the simulation shown below, the minimum principal stress is 8300 psi in the middle layer and 7550 psi in the surrounding formations. Pressure is 6500 psi in the middle formation, and 5500 psi in the surrounding formations. The screenshot shows the fracture at shut-in.


Initially, the fracture propagates radially within the middle zone. Once it reaches the surrounding low-stress layers, it preferentially propagates in these formations, leading to an ‘hour-glass’ shape. Also, the minimum principal stress is increasing with depth, which causes a general tendency for the fracture to propagate upward within each zone and causes a slight distortion of the hourglass shape.
The fracture mechanically closes in the middle layer quickly after shut-in. However, the mechanically closed fracture remains conductive, and so the wellbore has a hydraulic connection to the mechanically open fractures in the surrounding lower stress layers. Thus, the pressure transient observed at the wellbore is controlled primarily by the stress in the surrounding depleted layers, not the stress in the high stress layer in the middle.
DFIT Intersecting a Preexisting Depleted Fracture
In this scenario, the DFIT fracture intersects a preexisting, depleting hydraulic fracture. To create moderate height confinement, the problem is set up with a 40 ft thick layer with minimum principal stress equal to 8300 psi, and with surrounding layers that have stress 300 psi greater. The reservoir pressure is set to 7500 psi. A preexisting fracture – representing a hydraulic fracture formed during prior stimulation of a neighboring well – is placed at one side of the model. It is connected to a well that is producing at constant bottomhole pressure of 5500 psi. Production is performed for 40 days prior to the DFIT.
ResFrac solves the full poroelasticity problem (coupled equations of stress equilibrium, elasticity, and compatibility) to calculate the three-dimensional distribution of stresses induced by the depletion of the preexisting fracture. The Biot coefficient is set to 1.0.
The fracture formed during the DFIT intersects the depleted fracture. Subsequently, fluid drains out of the DFIT fracture into the depleted fracture. The results are shown below. The initial, depleting fracture is on the right and the fracture formed during the DFIT is on the left. The screenshot shows the fractures at shut-in.


Interestingly, the closure pressure in the transient is approximately 8300 psi, the same as the initial minimum principal stress in the middle zone. However, the apparent reservoir pressure in the transient is 5500 psi, corresponding to the pressure of the depleted preexisting fracture, not the initial reservoir pressure (7500 psi). This result contradicts the ‘rule of thumb’ prediction based on Equation 1. The pressure measured by the DFIT is 2000 psi depleted from the initial pressure (7500 psi to 5500 psi), yet the closure pressure is around 8300 psi, the same as the initial (non-depleted) minimum principal stress. How is this possible?
The lower panels in the figure above show the distribution of pressure depletion and the resulting induced stress change around the depleted fracture. The bottom right panel indicates that the decrease in the minimum principal stress around the depleted fracture is about 200 psi, only 10% the magnitude of the pressure drawdown. This may seem surprising, but it is not an erroneous calculation!
Equation 1 assumes that depletion is laterally homogeneous. In other words, if pressure in the entire formation was depleted to 5500 psi, then we would expect that Equation 1 would be valid, and the stress would decrease by 2/3-3/4 of the decrease in pressure. But in reality, pressure drawdown is strongly heterogeneous. Because of the low matrix permeability in unconventional formations, pressure depletion is localized around conductive hydraulic fractures. The size and shape of the region of depletion depends on the formation permeability, fluid viscosity, fracture geometry, and other parameters. The size and shape of the region of pressure depletion has a profound impact on the change in stress from depletion.
A classic paper on this subject is “The Effect of Thermoelastic Stresses on Injection Well Fracturing” by Perkins and Gonzalez (1985), SPE 11332. They discuss thermoelastic stresses induced by cooling, rather than poroelastic stresses induced by depletion. But poroelastic and thermoelastic stresses are mathematically equivalent in many aspects. With a ‘known’ distribution of pressure or temperature change, the calculation of induced stress is similar for thermoelasticity and poroelasticity.
Perkins and Gonzalez (1985) provide an analytical 2D solution for the stresses induced inside an elliptical region of uniform cooling (equivalent to an elliptical region of uniform pressure drop). For the special case of an ellipsoidal region, the induced stress change inside the ellipse is spatially uniform (this is not true in general, only because the region is ellipsoidal). The induced change in stress in the direction perpendicular to the long axis of the ellipse (equivalent to the induced change in normal stress on the fracture) is:

(2)
Beta is the linear coefficient of thermal expansion, E is Young’s modulus, and DeltaT is change in temperature. Equation 2 can be written for poroelastic stress change by replacing DeltaT with 'change in pressure' and replacing beta with a poroelastic modulus. The length of the long axis of the ellipse is a and the length of the short axis of the ellipse is b.
Here is the key point: Equation 2 shows that the induced stress tends to zero as the length of the short axis of the ellipse (b) tends to zero. In other words, if the zone of depletion around a depleting fracture is thin relative to its length, then the induced stress change normal to the fracture will be small, much less than predicted from Equation 1. If you think about it, this should begin to make intuitive sense. Initially, the zone of depletion is very narrow and confined near the fracture, and induced stresses are weak. Over time, as the zone of depletion grows larger, the induced stress changes become greater. In the limiting case that the entire formation is uniformly depleted, Equation 1 becomes valid.
In case you still aren’t convinced, here is another supporting calculation. On page 73 of the book “Thermoelasticity” by Witold Nowacki (1986), there is an analytical solution for the distribution of stresses outside a rectangular cuboid of uniform temperature change (equivalent to a rectangular cuboid of uniform pressure change). I wrote an Octave script to implement this analytical solution and plots of the result are shown below. The rectangle of cooling is oriented in the direction of the y-axis. The color contours show the relative change in x-axis stress (perpendicular to the long axis of the rectangle) caused by uniform pressure depletion in the rectangular region. In the first plot, the region of depletion is specified to be very thin. As you can see, the stress reduction along this thin rectangle is relatively small. In the second plot, the region of depletion is specified to be much thicker. The reduction in stress along the rectangle is greater.
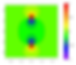

As an interesting trivia point, you might note that the stress is actually increased off the tip of the rectangle. If you think about it, you may realize that this is the exact opposite of the stress distribution created by an opening crack. Opening cracks create compression along their side and induce tension ahead of the tip. Here we have the opposite: a long skinny region of contraction inducing stress reduction along its sides and compression ahead of its tip. The concentration at the tip is a consequence of the equations of compatibility, which require the entire medium to strain in a way that smoothly accommodates the overall distribution of deformation.
The concentration of compression can be seen ahead of the tip of the preexisting fracture in the ResFrac screenshot above. This region of compression could hypothetically inhibit the propagating DFIT crack from reaching the preexisting fracture. But in practice, if the DFIT crack approached slightly from the side (rather than directly off the tip), it could bypass the region of stress concentration and then curve inward to intersect the depleting crack. Also, if production has occurred for long enough that pressure depletion propagates ahead of the tip, this could reduce or eliminate the concentration of compression.
Conclusions
The 2/3-3/4 ‘rule of thumb’ is only valid if depletion is laterally uniform within the layer. In unconventional formations, depletion is localized around conductive hydraulic fractures and is far from being laterally uniform. In this case, the induced stress change depends on the idiosyncrasies of the geometry of the region of depletion and changes over time. The induced stress changes from depletion may be much lower than predicted by the 2/3-3/4 ‘rule of thumb.’













